page 34
Note: the contents of this page as well as those which precede and follow, must be read as a continuation and/or overlap in order that the continuity about a relationship to/with the dichotomous arrangement of the idea that one could possibly talk seriously about peace from a different perspective as well as the typical dichotomous assignment of Artificial Intelligence (such as the usage of zeros and ones used in computer programming) ... will not be lost (such as war being frequently used to describe an absence of peace and vice-versa). However, if your mind is prone to being distracted by timed or untimed commercialization (such as that seen in various types of American-based television, radio, news media and magazine publishing... not to mention the average classroom which carries over into the everyday workplace), you may be unable to sustain prolonged exposures to divergent ideas about a singular topic without becoming confused, unless the information is provided in a very simplistic manner.
Let's face it, humanity has a lousy definition, accompanying practice, and analysis of peace.
There appear to be so many variables and so many models that can be used to analyze the dimensions of the peace/war phenomena, do we isolate ourselves from being overwhelmed by restricting which variables and which models are to be used? And though try as one might to apply some measure of polymathics to the topic, are there no finite answers to be obtained because we aren't even quite sure of how to define (the) words peace and war or whether they aren't mere metaphors themselves of something else?
On the other hand, if we leave our analysis open to multiple variables, are we displaying behavior to Don Quixote in that we ensure ourselves of always being able to find yet another adventure because we do not limit our imaginations? Is it possible to use a methodology of excursion by way of stating definitives that will provide an answer that can then be used as part of a list of examples compiled for applied restrictions which provide a result; thus providing an overall collective view? In other words, is the usage of restrictions in analysis a means by which we provide ourselves with a process of elimination? For example, if we use a set of definitive values and variables within a given type of research perspective (anthropological, biological, environmental, sociological, etc., causes), and they each render their own answers (or at least presumptions), do all of them provide a means of helping to assess whether any single or multiple avenues are more prosperous; or that all of them only provide a miniscule appreciation of that desired?
If our desire is to be able to stop war from ever occurring again, and/or making sure peace is permitted to evolve beyond its present formulation, and the type of analysis and/or application being used does not accomplish our goal, do we excuse ourselves and/or our efforts by claiming that the blame for deficiency is due to something other than our efforts? Or do we begin anew with another approach, perhaps in a different setting with those who share our interests? Then again, even if one were to have a definitive means of ending all wars forever, if the application of such required the assistance of others... yet most others are unable to grasp what it is you are trying to convey... will we give up or re-package our ideas in different mediums and venues so as to establish a social trend for others to become aware and convinced of the merits thereof?
Do we simply throw up our hands in frustration because "no one" listens, to the effect that one's ideas are not uniformly and universally accepted and adopted? Then again, we don't actually know how many people don't want to solve the problems of peace instead of simply managing them to the extent that such management requires a bureaucracy in which they and others might find possible employment. No less, due to the lack of peace and conflict resolution courses being taught in all grade levels, and the lack of such in social conversations, we might be inclined to think most people either don't think the topics are of value to be discussed, or the majority has independently concluded that there will never be "real" and lasting peace, but there will be war of one type of another because there is a general consideration that humanity is, by nature, a violent creature... at least human males... though human females have seemed to join the trend as expressed by the increased interest in sports competition, business competition, various beauty pageant competitions, academic competitions, scientific perspective competitions, etc...
And while some may claim that such competitions are not violent, they are in some instances what may be called "cutthroat" in that they are not concerned with whether one or more others suffers a loss of prestige, position, or pecuniary support... since such items may be that which are being sought by a person with a competing view... that is if they can persuade others in adopting a supportive role; either because they believe in the different perspective, and/or that they don't like another... or that differing competitive ideas are felt not to have any bearing in any case... but fill a void such as providing a researched academic topic that can be offered as a course that provides an educational institution an income from fees paid by students who are required to take the course... even if it is of little application outside the halls of academia.
In our search for answers we may find ourselves asking the simple question of whether peace and/or war are made by humans or discovered... in effect, where does "it" (as a dichotomy) or "they" (as separate entities) come from? And if made or discovered (intentionally and/or accidentally), why are they used? Has the pristine (original) "usage" been discarded or forgotten and the present usage is due to habit? Do they represent "proofs", axioms, or a fundamental logic of human contemplation? Will it require the adoption of a triple-patterned idea to supplant the peace/war dichotomy, such as a search for a third idea in which to answer all dichotomous-based physics issues discussed on page 25 of this series? With this perspective in mind, let us now turn to an article which asks if mathematics is discovered or created, and note the application of a triune philosophy as a model which attempts to provide a stepping stone that might help interested others in obtaining a higher grasp of the overall discussion and present to them the realization of a revelation:
October 12, 2015
Josh Wilkerson
By Dusty Wilson, Highline College
The following is from a talk given by Dusty Wilson at the ACMS Conference this past May. It is with his gracious permission that I am sharing it here. Here is a link to a previous talk given by Dusty on "Where does mathematics come from?"
Abstract
What is mathematics and is it discovered or invented? The Humanist, Platonist, and Foundationalist each provide answers. But are the options within the philosophy of mathematics so limited? Rather than viewing and describing mathematics in a mutually exclusive manner, each of these approaches includes components of truth from a greater triune philosophy of mathematics. This paper will briefly outline existing philosophies and then introduce an inclusive triune paradigm through which to explore fundamental questions about mathematics.
1 Introduction
My parents were hippies who were leery of traditional education. So out of a desire to both protect and also encourage questioning they put me into alternative public schools. These weren't edgy enough and so they allowed me to home-school junior high into high school. This put me on a fast track and I began community college during what would have been my junior year of high school. I jumped right into calculus and worked my way through differential equations. After two years I transferred to The Evergreen State College to continue my alternative education with an interdisciplinary liberal arts degree studying political science, literature, and mathematics. With such an eclectic background, I didn't have a clear direction following my bachelor's degree so I went on to graduate school in mathematics thinking, "If this doesn't work out, I can do something else later." While a graduate student I was given the opportunity to teach and my career path suddenly became clear. I was hired by Highline College right out of graduate school where I became the youngest tenured faculty member in College history.
While this makes me sound smart, it really means that I had a lot of growing to do as an educator and colleague. But I was in a supportive environment and by my eighth year I was firmly established as a teacher, in service, and in professional growth, and I generally felt that I knew my professional direction. In 2008 I attended a talk by a colleague [2]. The talk itself was on polling and statistics and not related to this paper. However in the midst of the lecture my coworker said, "I think math was invented by people, not discovered."
Is math discovered or invented? In all my non-traditional education as well as traditional community college and graduate studies and then continuing into the first eight years of my professional work, I don't once recall having asked myself the question, "Where does math come from?" But while this was the first time these ideas had ever registered in my mind, I have come to realize over the last seven years of study that I had subconsciously adopted a framework for understanding mathematics. As John Synge said, "[E]ach young mathematician who formulates his own philosophy — and all do — should make his decision in full possession of the facts. He should realize that if he follows the pattern of modern mathematics he is heir to a great tradition, but only part heir." [9, pp. 166] This certainly encapsulates my mathematical journey.
As I have come to have "full possession of the facts", I've learned that there are three main ways to explain the origin of mathematics. Within each of these broad categories there is a spectrum of nuance. Others have written compelling descriptions of this, but allow me to outline using broad strokes so that I may synthesize the field. The three broad views are as follows.
- Foundational philosophies: Mathematics is developed from axioms and definitions using logic.
- Humanistic philosophies of mathematics: Mathematics is invented by humans who are the source of math.
- Mathematical Platonism: Also called 'mathematical realism', this view holds that mathematics exists 'out there' to be discovered; perhaps owing its existence is to God, but perhaps not.
While some readers may recognize or be able to articulate their philosophy of mathematics, others may resonate with my story in that I was years into my career as a professional without realizing that I even had a view. I believed mathematics devoid of presupposition without even having the vocabulary to articulate my own presupposition about the field. So as I clarify the basic views available for later synthesis, I encourage you to ask yourself where these views match your training, intuition, and pedagogy.
2 The Foundational Philosophies
The first paradigm is that math is logic — this is the basis of the foundational philosophies: intuitionism, logicism, and formalism. If you do research on the philosophy of mathematics, these three views are described over and over again to the point that they nearly define the field.
The intuitionists such as Kronecker and Brouwer held that humans create the axioms of logic/mathematics and that we then manipulate these axioms to construct the theorems of mathematics in a constructivist manner. Because it stems from our work, the intuitionism shows existence by demonstrating a formula/algorithm/recipe to explain how each entity may be constructed. Because of this, intuitionists rejected proof by contradiction as well as the existence of an actual infinity. For them the source of mathematics was decidedly human. Or as Kronecker famously wrote, "God made the integers, all else is the work of men." [11, pp. 19]

The logicists movement was begun by Frege, reached its height with Russell and Whitehead, and concluded with Godel. They felt that the axioms of logic were self-evident truths that were known intuitively to the logician. They accepted the rules of logic apriori. In their effort to make solid their foundation, they held that some axioms were self-evident that are not so evident. Certainly the axiom of choice is on this list. Of the foundational camps, logicism was the most fully developed. For the logicist, the source of mathematics was beyond the human experience, self-evident, and discovered (albeit by a select few).
The formalists led by Hilbert were perhaps the largest group. They did not concern themselves with the source of the axioms but worked from these using every clever device they could devise. They had no issue with contradiction or infinity. Hilbert referred to math as a meaningless game. [1, pp. 21] The formalist didn't have a strong opinion about where mathematics comes from; after all, it didn't matter anymore than the source of Chess or Monopoly.
3 Mathematical Humanism
The second paradigm is mathematical humanism: all mathematics is somehow human in nature/origin. Unlike the foundational philosophies, the sub-categories are not as clearly defined. In part this is because mathematical humanism is more current and thus hasn't had as much time to mature. The spectrum within mathematical humanism that I will discuss ranges from a biology-brain model, to language, and ends with social constructivism. Of these, the idea that math is a language is probably the oldest while social constructivism seems most dominant among educators.
According to authors Lakoff and Nunez, our ability to perform abstract reasoning is biological. [8, pp. 347] Mathematics is ultimately grounded in experience. [8, pp. 49] It is effective because mathematics is a product of evolution and culture. [8, pp. 378] Mathematics doesn't have an independent existence. It is culture dependent and only exists through grounding metaphors. [8, pp. 3 356, 368] Consequently the philosophy of mathematics is the realm of cognitive science and not the domain of mathematicians. [8, pp. xiii] Where does mathematics come from? For these philosophers, the source of mathematics is biological and evolutionary and thus serves only an evolutionary purpose. . . which is to say it has no intended purpose.
Perhaps the most commonly held humanistic philosophy of mathematics is summed up in the phrase, "Mathematics is the language of science." This originates with Galileo who wrote: "[The universe] cannot be understood unless one first learns to comprehend the language and read the letters in which it is composed. It is written in the language of mathematics, and its characters are triangles, circles, and other geometric figures without which it is humanly impossible to understand a single word of it." [3, pp. 4] Today this phrase is most often used outside of university math departments because it defines mathematics through its applications and universities produce pure mathematicians (more akin to the formalists of the foundational movement). The basic premise of the view is that mathematics is invented as a way to describe discoveries in the natural world. Math isn't monolithic and unchanging because language changes. The strength of this view is that it seems to explain the perceived transcendence and beauty in math by tying it back to science.
Mathematics is something people do according to Reuben Hersh. [5, pp. 30] The philosophy of mathematics is the study of what mathematicians do. [5, pp. xii] The emphasis of social constructivism is on practice. As a practice there has been an evolution of mathematical knowledge. [5, pp. 224] This extends even to including proof itself. [5, pp. 6] As such, mathematics is a social construction. It draws on conventions of language, rules, and agreement in establishing truths. Mathematical knowledge and concepts change through conjectures and refutations. The focus is on creation rather than the justification knowledge. [5, pp. 228]
4 Mathematical Platonism
The third paradigm is mathematical Platonism (or mathematical realism) and is loosely based on the Plato's theory of forms and divided line. There is less explicitly written supporting Platonism (and much against). However many mathematicians are Platonists although not aware of it. Some know it and are reluctant to admit it because it seems mystical. Unlike the foundational philosophies and mathematical humanism, there is less written on the subtlety and nuances of Platonism. Thus the spectrum that I am about to describe is of my own creation (that is, unless I happened to get it from some abstract realm).
As given in Principia, Russell practiced what I dub, "finite mathematical Platonism." He began from a short list of self-evident “discovered” axioms. Then mathematics was built/created from these few eternal building blocks. This is a similar approach to that taken by Euclid. Finite Platonism gels nicely with the axiomatic method. It probably isn't a stretch to claim that the opti- 4 Mathematical Platonism/Realism Finite Axioms Countable Uncountable (All Truth) (Kitchen Sink) Figure 3: How much lies within the Platonic realm? mism following the "discovery" of Newton's laws stemmed from this same view: namely that the universe could be described by just a few simple laws. Today physicists are searching for the theory of everything . . . a few simple statements to describe all. In this view, very little is required of the mathematical form (which only contains a few statements) and much of the human mathematician (who massages the few givens into the body of mathematics). So where does mathematics come from? Well it begins with a few eternal truths and then is created by the mathematician.

The most widely held version of realism holds that all mathematical truth is found "out there" (in the Platonic form). This includes triangles, pi, and the golden ratio. It also includes every real number (yes, I know they are uncountable) and proofs big and small (think the proof of Pythagorean theorem vs. that of Fermat's Last Theorem). That is every true mathematical statement (regardless of how useful or elegant) can be discovered in the form. One author, Lakoff, calls this the romance of mathematics. [8, pp. xv]
Finally, the philosopher Alvin Plantinga affirmed what I call an uncountable mathematical Platonism. As a theist, he holds that math exists in the mind of God. He sees all mathematical entities as uncreated necessary beings whose existence is affirmed by God's nature. For Plantinga, God affirms the existence of all propositions, states, and possible worlds. But God affirms the truth of only some. [10, pp. 143] This is a kitchen sink view. That is everything exists "out there" to be discovered—true and false. But not all is true.
5 Mutually Exclusive Models?
In my reading, most authors want you to choose one and only one paradigm. They often use an elimination argument to justify their position. For example, a typical humanist's argument might be summarized: formalism is dead and Platonism requires God. Thus the only option remaining to us is humanism. The problem is that this assumes that: (a.) the discussed options are disjoint, (b.) that all the options are being considered, and (c.) that the premises are correct. I am going to focus on the first assumption: namely that the options are disjoint.
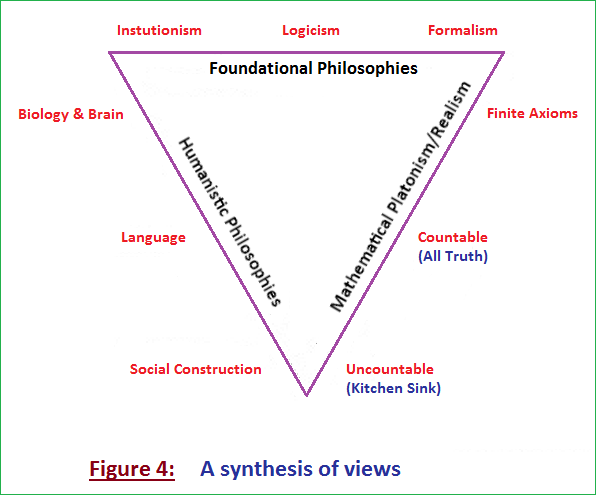
At first glance, Platonism, humanism, and the foundational views seem mutually exclusive (disjoint). If math is discovered “out there” then it can't originate within us. If it comes from within us, then it isn't a game we play, and certainly a meaningless game sounds nothing like the eternal truths of an 5 Humanistic Philosophies Mathematical Platonism/Realism Foundational Philosophies Intuitionism Logicism Formalism Biology & Brain Finite Axioms Language Countable (All Truth) Social Construction Uncountable (Kitchen Sink) Figure 4: A synthesis of views ideal realm. But perhaps this is a false trichotomy.
Consider the often told Indian parable of blind men trying to describe an elephant. One blind man feels a serpent, another a tree, and a third a spear. While these seem very different, we know that the legs, trunk, and tusks of an elephant are all part of the same animal. Could our seemingly mutually exclusive views of mathematics simply be appendages of a greater and more inclusive truth?
What I am proposing is a triune philosophy that envelops and includes much of a wide swath of the paradigms discussed. Whereas before, we saw three world views, each with its own nuances, now we envision mathematics on a higher dimension.
Trinity math
The key to this view is quite simple. Namely that the center of each of the three views represents the strength of the position. I dare say that many would agree that mathematics is a logical language we speak to describe abstract or immaterial truths.
To understand this view, it is insightful to think about what each paradigm sees as its greatest ideological adversary. We see this by comparing the center of each edge with its opposite vertex.
Example 1
Mathematics is a product of the neural capacities of our brains, the nature of our bodies, our evolution, our environment, and our long social and cultural history.
Authors Lakoff and Nunez explain their view and make it very clear who/what they view as their opposition. "Mathematics as we know it is human mathematics, a product of the human mind. Where does mathematics come from? It comes from us! We create it . . . Mathematics is a product of the neural capacities of our brains, the nature of our bodies, our evolution, our environment, and our long social and cultural history." [8, pp. 9]
They take on Platonism very directly. They write, "Human beings can have no access to a transcendent Platonic mathematics, if it exists. A belief in Platonic mathematics is therefore a matter of faith . . . There can be no scientific evidence for or against the existence of a Platonic mathematics . . . therefore human mathematics cannot be part of a transcendent Platonic mathematics, if such exists." [8, pp. 4]
Whether you accept their argument or not, it should be clear that they see the primary counterargument to their biology & brain explanation for the origin of mathematics as what they dub "The Romance of Mathematics." As they write, it's the stuff of movies like 2001, Contact, and Sphere. But while it initially attracted them to mathematics, they are now more enlightened. [8, pp. xv]
Example 2
Formalism vs. mathematics as the language of science—the debate between pure vs. applied mathematics.
The distinction we make between pure and applied mathematics is relatively recent. Are we standing on the shoulders of mathematicians or physicists — a good argument can be made for both. Prior to 1900, one can make the broad generalization that there was some pure mathematics but no pure mathematicians. But around the time when the foundational philosophies were being developed, this distinction was drawn. As G.H. Hardy wrote, "Pure mathematics is on the whole distinctly more useful than applied." [4, pp. 134] Taking this one step farther, the father of Formalism, David Hilbert is quoted as saying, "Mathematics is a game played according to certain simple rules with meaningless marks on paper." For the formalist, mathematics was certainly a language. However, it wasn't a language intended to communicate information outside of mathematics. Rather than being the language of science, mathematics was the language of mathematics.
The author Morris Kline wrote that most mathematicians have withdrawn from the world to concentrate on problems generated within mathematics. They have abandoned science. [7, pp. 278] Today mathematicians and physical scientists go their separate ways . . . mathematicians and scientists no longer understand each other. [7, pp. 286] Under the influence of formalism and the other foundational philosophies, mathematicians no longer speak the language of science.
Example 3
Mathematics is fallible and a social construction.
The social constructivists reject the narrow definition that math is logic. For example, the humanist Reuben Hersh is concerned with the edifice that remains in university mathematics departments. He believes that his philosophy recognizes the scope and variety of mathematics, fits into general epistemology and philosophy of science [note, science and not mathematics], is compatible with practice — research, application, teaching, history, calculation, and mathematical intuition. He also rejects certainty and indubitability as false and misleading. [5, pp. 33]
The opposition is clear: It's the foundational philosophies (primarily formalism as the most dominant view). Proof in particular is the opponent of this view. Hersh writes, "The trouble is, 'mathematical proof' has two meanings. In practice it's informal and imprecise. Practical mathematical proof is what we do to make each other believe our theorems. Theoretical mathematical proof is formal. It's transformation of certain symbol sequences according to certain rules of logic." [5, pp. 49] The only reason to believe in mathematics is—it works! [5, pp. 213] There is no infallibility. [5, pp. 215]
Hersh wants to redefine mathematics as fallible and a social construction. As such he must take on the establishment. And the power brokers in mathematics hold the foundational view that math is logic and as such is pure and unchanging.
Before further sharing what I call the triune philosophy of mathematics, it's important to recognize that ideas have consequences, and that this remains a truism in the philosophy of mathematics as it is elsewhere. The way we answer "Where does math come from?" impacts research and education. Given my own background as an educator, I'd like to say few words on education.
Example 4
The philosophy of mathematics and its influence on education.
Nicolas Bourbaki is the collective pseudonym under which a group of mathematicians wrote a series of books with the goal of grounding all of mathematics in set theory. Their approach is similar to that of the formalists. The manifesto of Bourbaki has had a definite and deep influence. In secondary education the new math movement corresponded to teachers influenced by Bourbaki. "The devastating effect of formalism on teaching has been described ... [5, pp. 238]" through books like Why Johnny Can't Add. [6]
Today we can see the influence of each major paradigm through the competition between teaching through a discovery method, cooperative learning, and skill based manipulations. Given the massive fiscal investment in mathematics education in the U.S., finding the perfect pedagogy is somewhat of a holy grail. But what if our issue is having too limited a view on mathematics? While I don't claim to be an expert on human cognition or learning, I postulate that a pedagogy that incorporated aspects of all three major philosophies would be more attractive to the next generation of students. If you will, it's almost a philosophical parallel to teaching to multiple learning styles.
6 A Synthesis of Views
If the three major branches of the philosophy of mathematics are not mutually exclusive, it is possible that a broader, more inclusive, philosophy of mathematics exists. Is mathematics invented or discovered — yes. I'm proposing a view that incorporates the strengths of each paradigm but that comes with some ambiguity — what I am calling, "A triune philosophy of mathematics."
Just to clarify, this essay isn't intended to end a discussion but rather to begin a conversation. What is good? What arguments are logically sound? What passes the experiential sniff test? This conversation is going to force us to go much deeper into the details than this paper has allowed. And as Whitehead and Russell learned full well, the devil is in the details.
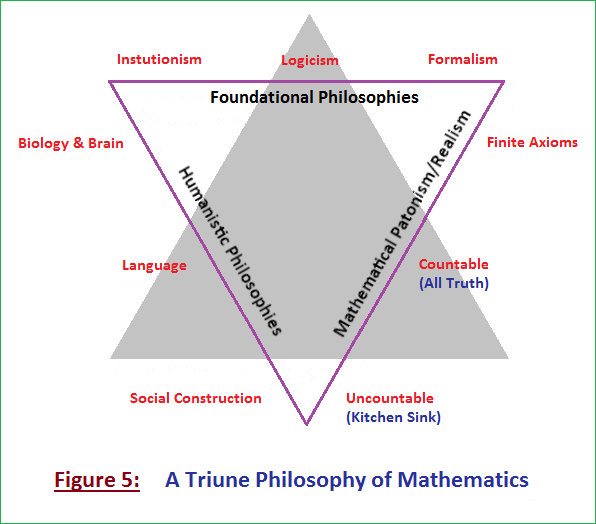
Figure 5: A Triune Philosophy of Mathematics
For me, this was the image that first opened my eyes to a triune philosophy of mathematics. It incorporates the greatest strengths of each paradigm inside a single figure. There is a common practice of mathematics between philosophies. That is, our old friends from calculus and algebra haven't changed—the integral and derivative are calculated the same way whether discovered, invented, or based on the axioms of logic.
One powerful aspect of this model is that it gives a place to look for counter-arguments. That is, the center of each edge of the original triangle is strong while its vertices are potential weaknesses. As we look to certain places to find counter examples to prove/disprove a mathematical claim, this gives us a direction to look to substantiate/discount philosophical arguments.
Bear in mind that the distinctions I am making are tentative. That is the vertices of the new solid triangle (triune math) could shift to include more/less of the gray triangle. For that matter, one might argue that these are not triangles at all, but that there are many more sides on each figure. But while I acknowledge that this is a legitimate objection and requires serious consideration, it isn’t my hypothesis.
For the humanist, the source of truth must come from within the cosmos. The Platonist says math resides outside the material world. The foundationalist says that math is from self-evident axioms and doesn't bother to justify their existence. Going back to the parable of the blind men and the elephant, there was a clear source for higher knowledge (namely the elephant). If this triune philosophy more fully describes the nature of mathematics, then it too is likely grounded in a greater rationale.
I believe mathematics is firmly grounded in the triune God of orthodox Christianity. Following in the footsteps of Kepler, Newton, Euler and countless others, I believe that there are aspects of mathematics that go beyond the physical world:
- Mathematics is logical and self-existent because it is part of the nature of a logical and self-existent God.
- Humans create and speak the language of mathematics as image bearers of one who walked among us.
- We can discover eternal transcendent truth because the spirit of God speaks to each one of us.
For some the very mention of God may be enough to discredit this whole triune philosophy of mathematics. For others, the selection of a specific God may be too much. I accept this critique but challenge you: Is there any existing philosophy of mathematics that fully describes the marvel and practice of mathematics? If not, could there be a greater elephant in need of description? If so, what is its size and shape?
Conclusion
So far as I know, this triune philosophy of mathematics is a new idea (perhaps discovered, perhaps invented). This essay marks the first time it has been shared in print. It's quite possible that I will soon find out the importance of tenure as this could be the last essay I ever write. Jests aside, I anticipate next steps in two directions. The first is in answering the likely objections that this paper will receive. The second is in fleshing out the details wherein the truth most likely lies.
Is it worth it? Yes, ideas have consequences and we have gone too many years under the allusion that mathematics is a field devoid of presuppositions. This introduction to a triune philosophy of mathematics should bring this out in the open. Something needs to change in mathematics and I propose that it is how we view and understand where mathematics comes from.
References
[1] E. Bell. Mathematics: Queen and Servant of Science. Bell, London, 1952. [2] H. Burn. Polling: When mathematics meets the real world. In Highline College: Science Seminar, 2008. [3] G. Galilei. The Assayer. unknown, 1623. [4] G. Hardy. A Mathematician's Apology. Cambridge, Cambridge, 1940. [5] R. Hersh. What is Mathematics, Really? Oxford, New York, 1997. 10 [6] M. Kline. Why Johnny Can't Add. Random, New York, 1974. [7] M. Kline. Mathematics: The Loss of Certainty. Oxford, New York, 1982. [8] G. Lakoff and R. E. Nunez. Where Mathematics Comes From. Basic, New York, 2000. [9] J. Nickel. Mathematics: Is God Silent? Ross House, Vallecito, 2001. [10] A. Plantinga. Does God Have A Nature? Marquette, Milwaukee, 1980. [11] H. M. Weber. Obituary for leopold kronecker. unknown, 2:5—31, 1891/2.
Source: A Triune Philosophy of Mathematics
At this point, let us ask if mathematics is real or is it a figment of our imagination rendered into symbols which helps us to connect, correlate, and condition mental processes to a type of activity that is described as a formula because it permits the human ego to thump its chest, so to speak? Is there a triune godhead (father, son, holy spirit), or is the value "three" a portrayal of an environmental event having impressed itself onto our psyche and physiology... as well as being an environmental event that is undergoing a decay... which affects the "three" pattern? Is the "three" pattern an expression of "fusion" related to the combining of separate but connected environmental events? For example, the long history of the Sun's three "moments" called dawn-noon-dusk would have irradiated the early Earth and its different-than-today environment, in a stroboscopic-like fashion due to an accelerated rotation of the Earth. But as the Earth's rotation continues to slow down, and the Sun heads towards a burnout with an eventual expansion, the three "moments" will, in essence, "fuse together" causing a three-to-one ratio such as we presently see in the concept of the "three persons in one godhead" idea. Similarly, we see a "three to one" ratio already present in DNA and RNA in that each has the three same amino acids (adenosine-cytosine-guanine), and one that differentiates them: Thymine for DNA and Uracil for RNA. No less, we might describe the three branches in one government as a primitive variation since there is an inclination to develop a "stand-alone" peoples Legislative branch that will effectively enhance the checks and balances provision. There are numerous other examples of which only a few need be mentioned, such as the usage of three fingers to hold one pen or pencil, three colors in one flag, three colors in one street light, three vending machine coins (nickels-dimes-quarters) to one paper denomination (dollar bill).
Because we are beginning to venture into another area of consideration to be applied to the peace/war discussion, let us take it up on the next page.
Date of Origination: Saturday, 11-Feb-2017... 10:02 AM
Date of initial posting: Thursday, 23-Feb-2017... 11:00 AM
Updated posting: Friday, 6-April-2018... 12:46 PM